Print this chapterPrint this chapter

Differential and Integral Calculus

7. Area

Area in the plane

We consider areas of plane sets bounded by closed curves. In the more general cases, the concept of area becomes theoretically very difficult.

The area of a planar set is defined by reducing to the areas of simpler sets. The area cannot be "calculated", unless we first have a definition of "area" (although this is common practice in school mathematics).

Starting point

The area of a rectangle
The area of a rectangle is base \(\times\) height: \[A=ab.\]

A rectangle
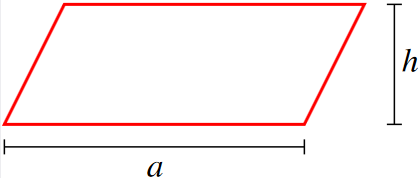
Definition: Area of a Parallelogram

The area of a parallelogram is base \(\times\) height: \[ A=ah. \]


parallelogram
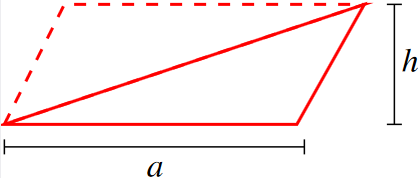
Definition: Area of a triangle

The area of a triangle is (by definition) \[ A=\frac{1}{2}ah. \]


triangle

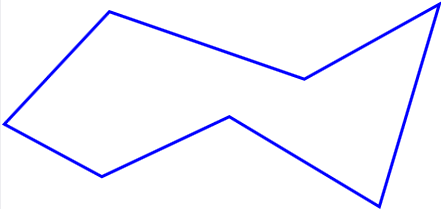
Polygon

A (simple) polygon is a plane set bounded by a closed curve that consists of a finite number of line segments without self-intersections.

polygon
Definition: Area of a polygon

The area of a polygon is defined by dividing it into a finite number of triangles (called a triangulation of the polygon) and adding the areas of these triangles.


triangulation
Theorem.

The sum of the areas of  triangles in a triangulation of a polygon is the same for all triangulations.


General case

For a plane set \(\color{red} D\) bounded by a closed curve we can construct inner polygons \(\color{blue}P_i\) and outer polygons \(P_o\): \(\color{blue}P_i\color{black} \subset \color{red}D\color{black}\subset P_o\).

A bounded set \(D\) has an area if for every \(\varepsilon >0\) there is an inner polygon \(P_i\) and an outer polygon \(P_o\), whose areas differ by less than \(\varepsilon\): \[ A(P_o)-A(P_i)<\varepsilon. \] This implies that between all areas \(A(P_i)\) and \(A(P_o)\) there is a unique real number \(A(D)\), which is (by definition) the area of \(D\).

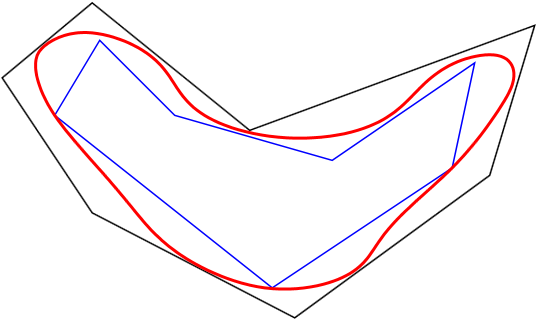
Inner and outer polygons

A surprise: The condition that \(D\) is bounded by a closed curve (without self-intersections) does not guarantee that it has an area! Reason: The boundary curve can be so "wiggly", that it has positive "area". The first such example was constucted by [W.F. Osgood, 1903]:

Wikipedia: Osgood curve

Example

Derive the formula \(A=\pi R^2\) for a circle with radius \(R\) by choosing regular inscrided and circumscribed \(n\)-gons as inner and outer polygons, and let \(n\to\infty\).

The solution is a voluntary exercise, where you need the limit \[\lim_{x\to 0}\frac{\sin x}{x} = 1.\] Hint: Show that the inscribed and circumscribed areas are \[ \pi R^2\frac{\sin (2\pi/n)}{2\pi/n} \ \text{ and }\ \pi R^2\frac{\tan \pi/n}{\pi/n}.\]